Lenses, Mirrors and Optics

Telescopes come in two basic types: refracting telescopes (using a lens as its main element: 1, 2, 3 ) and reflecting telescopes (using a mirror as its main element: 1, 2, 3). Simple ray tracing techniques exist for determining for a given optic (either a lens or a mirror) where an image forms, given an image distance.  The method is illustrated in the diagrams below.
 

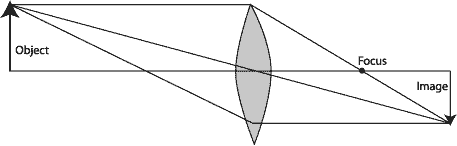Geometric optics ray tracing for a thin lens.  Given the focal distance f, and the  object distance do, one can determine the distance to the image di, geometrically. The rules are: parallel rays go through the focus, and rays through the center of the  lens do not bend.

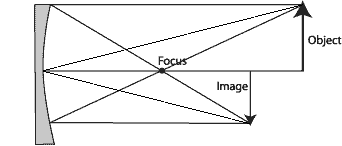Geometric optics ray tracing for a mirror works the same way.  The rules are:  parallel rays go through the focus, and rays that hit the center of the mirror  bounce off symmetrically.

The relationship between focal distance f, object distance p, and image distance q, is given by the formula:

1/f = 1/p + 1/q

which can be verified as consistent with the above rules through simple geometry (homework problem 6.2). Another important formula is the lens-maker's formula:

1/f_l = (n_l - 1) (1/R₁ + 1/R₂)

where n_l is the index of refraction at wavelength l, and R₁ and R₂ are the radii of curvature of the lens (negative radius for a diverging lens). Note that this formula assumes the lens is in a vacuum (works approximately for air). If the lens is in a medium with index of refraction n₀, then the first term in parentheses on the right should be (n_l - n₀)/n₀. Note that the index of refraction depends on wavelength (see http://refractiveindex.info/), so in general a lens will have a different focal length for red light (l ~ 750 nm) than for blue light (l ~ 450 nm). This causes chromatic aberation, which can be combatted by using an achromatic doublet, or an apochromatic triplet lens. An important property of mirrors is that all wavelengths are reflected the same, so mirror-based telescopes do not suffer from chromatic aberation.

An important number for a telescope's main lens or mirror (called an objective), is its f ratio, which is simply the ratio of the focal length to the diameter D of the objective

f ratio = f/D.

In the above diagrams, the f ratio is about 1, but for optical telescopes it is more typically about 10.  (For radio telescopes, it is usually less than 1, typically 0.4.  Why do you think that might be?)

The arrows in the above drawings represent an extended object, which covers some angular distance in front of the lens.  If this were the Moon, say, it would cover 1/2 degree in the sky.  When we make an image, it also covers some angular distance from the lens, and if we place a detector there (say a piece of photographic film), the image will have some linear size.  Now, the Moon can be considered to be at infinity (that is, d_o is much larger than d_i), so the image of the Moon will occur at the focal distance f (which is why it is called the focus).  In this case, the linear size per degree of angular size of the image at the image plane (called the plate scale) is simply

s = 0.01745 f

where the constant 0.01745 is just the number of radians in a degree, and f is the focal length of the objective.  The linear size of an image will be sa, where a is the angular size of the object in degrees.

Example:
Say we have a telescope of 8 inch aperture, and f ratio of 10 (also referred to as f / 10).  How large will the Moon's image appear on a piece of photographic film at the focus?

The focal distance f is 80 in, so the image will be

sa = 0.01745 a f = 0.01745 (1/2 degree) (80 in) = 0.7 in

You will often hear someone ask, "What power is that telescope?".  This is a relatively meaningless question, and indicates someone who does not understand the principles of optics.  Do not be one of these people!  Their confusion is understandable--low-end telescopes are often advertised by their "power,"  by which is meant their magnification factor.  You will never see a true astronomical telescope advertised this way.  What is really important is the light-gathering power of a telescope, not its magnification.  The light-gathering power is important, because this is what limits how faint an object one can see.  The larger the area of the objective, pD²/4, the larger the amount of light gathered.  The light-gathering power is not a fixed number, but is expressed in comparison with another optical system, say your eye.  Your pupil has a maximum size of about 0.5 cm, so the light-gathering power of an 8" telescope (diameter 20.3 cm) is

LGP = (20.3/0.5)² = 1650.

The main function of the telescope is to take all of this light and concentrate it into your eye (or an equivalent camera). In this case, a telescope would gather 1650 times as much light as your naked eye. A second important measure of a telescope also scales with its aperture--the resolving power.  This depends on the wavelength of light that we are trying to image, and is given in angular units, arcseconds, by

RP = 1.22 (206265 l/D) arcsec

where the constant 206265 is the conversion factor from radians to arcseconds.  The factor of 1.22 is due to the fact that the aperture is circular.  For a rectangular aperture the factor would be 1.0, but grinding rectangular lenses is not easy!

Example
What is the resolving power of our 8" telescope in the optical (l ~ 500 nm)? 8" is 20.3 cm, or 0.203 m, so the resolving power would be:

RP = 1.22 (206265 l/D) = 1.22 (206265)(5x10^-7 m/0.203 m) = 0.62"

Note, however, that the atmosphere usually limits the resolution to about 1", so having a telescope much larger than 8" does not help in resolution unless we either are above the atmosphere or can correct for the atmosphere.  A larger telescope does, however, help in light-gathering power.

What about magnification?  As it turns out, any telescope can be any magnification power!  It does not depend at all on the aperture, or size, of the telescope.  The magnification only depends on the ratio of the focal length of the objective f and the focal length of the eyepiece f_e:

MP = f / f_e.

Common eyepieces for telescopes are 26 mm and 12.5 mm.  An f/10 telescope of 8" aperture (203 mm) would provide corresponding magnifications of 78X and 162X for these two eyepieces.  However, you could get the same magnifications with a 2" telescope simply by using eyepieces of 6.5 mm and 3.1 mm focal length.  This is why asking what "power" a telescope has is not too meaningful.  Any telescope can have any power, depending on the eyepiece you choose.

However, there is an important relationship between light-gathering power and magnification, which makes higher magnifications useless for small telescopes.  Think of the objective as gathering light, and the eyepiece as spreading it out again.  Although you can spread the light out indefinitely with smaller focal length eyepieces, this reduces the brightness of the image until you reach a point where you cannot see it any longer.  This happens sooner, of course, for a smaller telescope, which gathers less light in the first place.  If you want to observe a faint, extended nebula, smaller powers are best (you see more of the sky at once, and the nebula is brighter).  If you want to observe a brighter object, like the Moon or a planet, you can often get away with higher power.  However, there is no point magnifying the image beyond the resolution of the telescope (or the atmosphere), since you will just magnify the distortions.

Three types of detectors have historically been used in optical astronomy:

• Photographic Film
• Photomultiplier Tubes
• Charge-Coupled Devices (CCDs)

The last type, the CCD, has for most applications replaced the other two (even in ordinary photography, with the rapid advances in digital cameras).  One reason is "quantum efficiency" or QE.  This expresses the ability of the detector to respond to photons, or light-quanta.  The human eye has a QE of about 1%, meaning that for every 100 photons that fall on our retina, only one is detected.  Photographic film has a similar QE, about 1%.  Photomultipliers have an efficiency between 10 and 20%.  In contrast, CCDs have much higher efficiencies, close to 100% in the red region of the spectrum.

Another reason for the increasing importance of CCDs is the large form-factors now available--up to 10 million pixels (e.g. KAI-11002 chip, 4008 x 2672).  It is common in astronomical detectors to place multiple CCD chips side by side for extremely large format images (e.g. the SDSS camera, LSST camera).

CCDs have other advantages as well.  They are linear devices, so that they give a precise measure of the number of photons falling on each pixel, over a very large range in brightness.  Ultimately, however, the pixels (quantum wells) will fill up, and the device saturates.  The electrons then spill over into neighboring pixels.  The image below shows an example of such saturation.

Two Sun-grazing comets plunge to their fiery death
into the Sun.  The image of one of the comets has
saturated the CCD camera, and its light spills into
neighboring pixels, making a horizontal line artifact.

CCDs also have the advantage of being purely digital, so they can be controlled easily with computers, and their data can be manipulated digitally.

Photons obey Poisson statistics, meaning that the arrival of photons into a given pixel is a random process, but always positive (there are no negative photons).  The statistical fluctuations in the mean number of photons <N> have a standard deviation given by s = <N>^1/2.  Thus, the signal to noise ratio is

S/N =  <N>/s = <N>^1/2.

Now, the mean number of photons detected by a CCD pixel will depend on the photon flux f_p (photons per second) x the integration time Dt x the quantum efficiency, so the signal to noise becomes:

S/N = (QE f_pDt)^1/2.

For a given quantum efficiency, we can increase the signal to noise by increasing the integration time.  However, to double the S/N, we must increase the integration time by a factor of 4.

The NJIT observatory has a computer-controlled 10" Meade LX200-GPS telescope.  We can use this telescope with an SBIG STL-1301 CCD camera for "deep-sky" imaging, or use a simple webcam for planetary and lunar imaging.  The telescope is an f/10 system, and the two CCD cameras have the following specifications:

Some CCD Camera Specifications

CCD	Kodak KAF-1301E	TouCAM II VGA CCD
Pixel Array	1280 x 1024 pixels 20.4 x 16.4 mm	640 x 480 pixels 3.58 x 2.69 mm
Total Pixels	1,310,720	307,200
Pixel Size	16 x 16 microns	5.6 x 5.6 microns
Full Well Capacity (NABG)	~120,000 e-	?
Dark Current	0.5e¯/pixel/sec at -30° C	?

What is the image scale of the KAF-1301E CCD (arcsec/pixel)?

The focal length is f = (f ratio)D = 10*10 in = 100 in. <= note these are inches!
The plate scale is s = 0.01745 f = 1.75 inches/degree.
One pixel is 16 microns, so this corresponds to an image scale of

(16 x 10^-6 m/pixel)(3600"/degree)/[(1.75 in)(2.54 x 10^-2 m/in)] = 1.29"/pixel

What is the field of view of the CCD camera, in arcminutes?

The chip has 1280 x 1024 pixels, and each is 1.29", so this becomes 1659" x 1321", or in arcminutes: 27.6' x 22.0'.

Will the Galilean moons of Jupiter fit into the field of view when Jupiter is at opposition?

At opposition, Jupiter is 4.2 AU from Earth, and is 71,400 km in radius.  This corresponds to an angular size, in arcsec, of 206265 (R/d) = 23".  The outer-most Galilean moon is Callisto, at 26.6 Jupiter radii, or 618".   This is smaller than the CCD field of view, so Jupiter and its moons will fit comfortably in the field of view. The planet would cover about 36 pixels of the image.

A sampling of images taken by students with this system can be found here. Note that there is a whole host of other issues involved with taking good images, involving background sky brightness, flat-fielding, and compensation for image motion.

We use the webcam for taking images (and movies) of the Moon and planets, because it has a far smaller plate scale (i.e. produces more magnified images). If the weather cooperates, we will have an imaging session this week.

Spectrographs are used to split the light from an object into its separate wavelengths, or colors.  One can determine the temperature of an object, for example, from the shape of its blackbody spectrum.  In addition, many objects emit spectral lines (narrow regions of the spectrum that are brighter than the background--emission lines; or darker--absorption lines).  By identifying these lines we can determine what the object is made of, and by measuring them we can determine the temperature, density, and speed (through the doppler effect) of the objects.  The spectrograph is an important tool of Astronomy.

The most common type of spectrograph is the grating spectrograph, which uses a diffraction grating (basically a set of extremely closely-spaced ruled lines).  Diffraction at the edges of each space between the lines causes the light to be split into its component colors, and interference of the light from each set causes the spectrum to be split into "orders".  For a simple grating, higher orders are fainter, but the light into a particular order can be increased by "blazing" the grating (kind of a saw-tooth profile) at the appropriate angle.

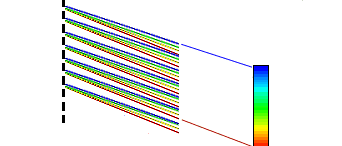
Diffraction into a high order.  In the direction shown by the blue lines,
the blue light interferes constructively because the beams all arrive at
the distant screen after having traveled distances different by an integral
number of wavelengths.  In the direction shown by the red lines, blue
light will arrive after traveling non-integer multiples of the wavelength,
so the light interferes and the intensity drops to near zero.  However, red
light arrives after traveling distances different by an integral number of
red wavelengths, so the red intensity is high in that direction.

As shown above, the direction for constructive interference into order m is given by

sin q = ml/d,

where d is the spacing between ruled lines on the grating. 

The important formulas and quantities for telescopes are:

• Image formula                1/f = 1/d_o + 1/d_i
• Lens maker's formula     1/f_l = (n_l - 1) (1/R₁ + 1/R₂)
• f Ratio                             f ratio = f/D
• Plate scale                     s = 0.01745 f
• Light gathering power   LGP = (D/d_eye)²
• Resolving power           RP = 1.22 l/D (radians)
• Magnification factor       MP = f / f_e

Another important formula is Signal to Noise ratio for CCDs:

• S/N = (QE f_pDt)^1/2.