Atomic Structure

To understand the origin of spectral lines, we must examine the internal structure of the atom.  In particular, we will start with a simplified model called the Bohr model of the atom, since it gives surprisingly good results despite its rather extreme over-simplification.  Bohr set out to explain

• location of spectral lines of hydrogen (Balmer series)
• Johann Balmer measured the wavelengths of this series of lines from atomic hydrogen and found that they fit the relation
1/l = R_H ( 1/4 - 1/n²)
where R_H is the Rydberg constant R_H = 10.96776 mm^-1.
• Using n = 3 gave the Ha line wavelength,  n = 4 gave Hb, and so on.

The hydrogen line spectrum, with the pattern of Balmer lines that Bohr set out to explain.

In the Bohr model, the nucleus is considered to be a point mass located at the center of the atom, while the electrons move in circular orbits around it, something like the solar system with planets moving around the Sun.

The positive charge of the nucleus and the negative charge of an electron give the electrostatic attraction force required to keep the electron in uniform circular motion.  Bohr's Postulates:

• Only a discrete number of orbits is allowed to the electron (only those with angular momentum that is an integral multiple of h/2p) and when in these orbits the electron cannot radiate.
• Radiation of a discrete quantum of energy is emitted or absorbed only when the electron jumps from one orbit to another, and the energy of the radiation equals the energy difference between the orbits.

The circular orbits postulated for the electrons around the nucleus of the atom means that there must be a central force (centripetal force) mv²/r provided by the attraction between the negatively charged electron and the positively charged nucleus.  This attraction is the electric force between two charges (the Coulomb interaction), whose magnitude is
F = (4pe_o)^-1q₁q₂/r² = (kZe)e/r²=mv²/r            (1)
where for convenience we use k = (4pe_o)^-1= 8.99 x 10⁹ N m²/C².  Recall that the angular momentum is mvr, and so Bohr's postulate about angular momentum is equivalent to:
mvr = nh/2p                                                  (2)
Combining equation (1) with (2), we can solve for r, the radius of the orbit, as
r = a_on² = n²(h²/4p²me²kZ).                                      (3)
where a_o= 5.29 x 10^-11 m is the Bohr radius for the ground state of the hydrogen atom.

Looking at the meaning of this equation, it says that electrons are allowed only orbits of certain (quantized) radii, where n = 1, 2, 3...     We will look at this further in a moment.

Associated with these quantized orbits is a quantized energy.  Total energy is the sum of kinetic energy and potential energy, E = K + U.  We are free to choose the zero of potential energy any way we wish, since only differences in potential energy are important.  We will take the zero of potential energy at infinity.  The potential energy difference is just the negative of work, or force times the displacement, so the potential energy of an electron inside an atom is

 

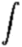		r	kZe2 dr
U =				= - kZe2/r
		inf	r2

so that the total energy is
E = 1/2 mv²-kZe²/r
and using equations (1), (2) and (3) above, after some manipulation we find the quantized energy
E(n) = -(2p²me⁴k²Z²)/n²h².
Note that the total energy is negative, which means that the electron is bound, and that as n -> infinity, E ->0.  The number n is called the principal quantum number.

The quantity (2p²me⁴k²Z²)/h² is a constant that depends only on fundamental physical quantities,

R' = (2p²me⁴k²)/h² = 13.6 eV.
The electron-volt (eV) is a unit of energy (the energy an electron would gain after acceleration through a potential of 1 volt). 1 eV = 1.602 x 10^-19 J.  Using the symbol R' for this constant, the total energy equation becomes extremely simple:
E(n) = -R' Z²/n².

Photons are "light particles," but light, of course, also has wave characteristics.  These two different views, wave vs. particle, are related in the expressions for photon energy (E = hn) and photon momentum (p = h/l).  de Broglie postulated that these photon relationships also hold for massive particles, and that a frequency and wavelength could be derived from:
• n= E/h
• l= h/p
where E and p are the classical energy and momentum of the massive particles.  This has been verified by many experiments, and is called wave-particle duality.  Related to this is the Heisenberg Uncertainty Principle, which has two forms:
• Dx Dp ~ h/2p
• DE Dt ~ h/2p

The Bohr model of the hydrogen atom (Z = 1) gave us the following results:

The angular momentum, l, was postulated to be quantized according to

l = mvr = nh/2p. where n is the principle quantum number.

The radii of allowed electron orbits were also quantized according

r = a_on² = n²h²/(4p²ke²m)

where a_o= 5.29 x 10^-11m is the Bohr radius.

The energies of the orbits were likewise quantized according to

E_n = -R' [1/n²]

where R' = 13.6 eV is a constant.

The difference between energy levels (orbits) corresponds to a wavelength

1/l_ab = n_ab/c = (E_b - E_a)/ch=R_H[1/n_b²-1/n_a²]

where R_H= 10.96776 mm^-1 is the same Rydberg constant that was measured experimentally by Rydberg.

One way to see why the angular momentum should be quantized is to consider the electron as a wave.  As de Broglie showed, particles exhibit wave characteristics with wavelength given by

l = h/p = h/mv

What if the lowest orbit corresponded to one wavelength of such an electron wave?  The circumference of the orbit would correspond to a wavelength, so

2pr = l = h/mv  => l = mvr = h/2p.

The next higher orbit would correspond to two electron wavelengths, and the nth orbit to n electron wavelengths, so in this way we naturally find l = nh/2p.  If the orbit corresponded to a non-integer multiple of the electron wavelength, the electron wave would overlap and interfere with itself.

Here is a scale model of the orbits of the hydrogen atom, with the radii getting further apart according to n²:

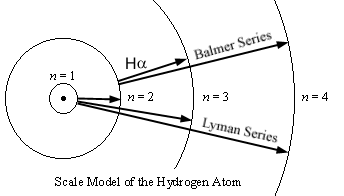
However, despite the orbits getting ever farther apart in space, they get closer together in energy according to 1/n².  Any energy level diagram is shown below:

We noted earlier the relationships:

Dx Dp ~ h/2p
DE D t ~ h/2p

Let us now show some effects that come from them.  The n = 2 level of hydrogen lives for a very short time, only about 10^-8 s.  That is, an electron in the first excited state will, after about 10^-8 s, spontaneously emit a photon of energy 10.2 eV and go back to the ground state (emitting a Lyman a photon).  The second relation, above, means that the photons emitted have an energy uncertainty of

DE ~ h/2pD t = 1.05 x 10^-19 erg  =>  Dl ~ l²/2pD t c = 0.014 mA

This uncertainty in the wavelength shows up as a broadening of the spectral line (natural broadening).

Similarly, we can ask what is the uncertainty in speed that is required of an electron that is confined in a hydrogen atom (in the ground state orbit, with Bohr radius a_o= 5.29 x 10^-11 m).
Example: Imagine an electron comfined within a region of space the size of a hydrogen atom.  What is the minimum speed and energy of the electron, estimated using the Heisenberg uncertainty principle?  We know Dx ~ a_o= 5.29 x 10^-11mso

Dp ~ h/2pa_o = 6.62 x 10^-34 J s/2p(5.29 x 10^-11m) = 1.99 x 10^-24 kg m/s.

This is the uncertainty in the momentum, which is about equal to the minimum momentum p_min that the electron has in order to be confined within the atom.  Now the momentum is just p = mv, so the momentum, so the minimum velocity is

v_min = p_min/m_e = 1.99 x 10^-24 kg m/s / 9.1 x 10^-31 kg = 2.19 x 10⁶ m/s.

The corresponding minimum kinetic energy is

K_min = 1/2m_ev²_min= 2.18 x 10^-18 J

which may seem like a small energy, but when converted to electron volts, this is

K_min = 2.18 x 10^-18 / 1.602 x 10^-19 J/eV = 13.6 eV!

This is exactly the energy of an electron in the ground state of the hydrogen atom!  Later we will see that this subtle quantum effect is responsible for supporting the tremendous gravitational weight inside a white dwarf star.